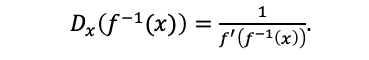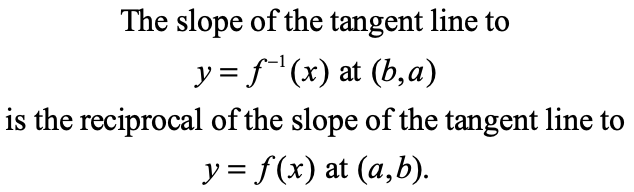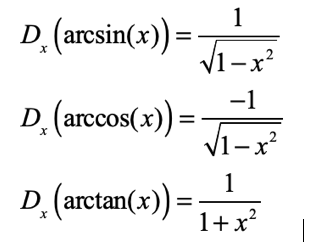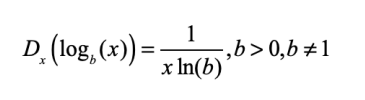Standard D05: Chain Rule + Advanced Techniques:
Objectives & Resources
1. Understand that the derivative of a function is defined as the limit of a difference quotient and can be determined using a variety of strategies, including knowledge of inverse function relationships and “implicit differentiation.” [EU 2.1]
2. Inverse Functions & Derivatives: Know that the chain rule can be used to find the derivative of an inverse function, provided the derivative of that function exists. [EK 2.1C6]
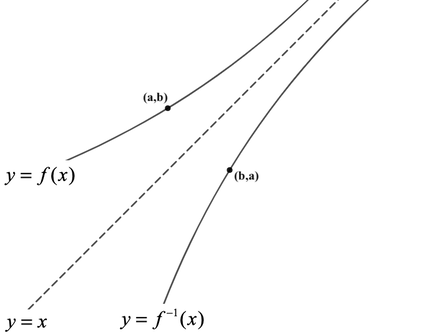
a. Know and understand the equation that relates the derivatives of a function and its inverse (see below).
a. Know and understand the equation that relates the derivatives of a function and its inverse (see below).
Use your knowledge of inverse function relationships!
|
EXPLORATION
Desmos: Derivatives of Inverse Functions |
b. Know the derivative rules for the inverse trigonometric functions.
c. Know the derivative rule for the general logarithmic function.
Know these derivative rules. Flash cards might help!
|
EXPLORATION Desmos: arcsine
|
EXPLORATION Desmos: arccosine
|
|
EXPLORATION Desmos: arctangent
|
EXPLORATION Desmos: general logarithmic function (base b)
|
3. Implicit Differentiation: Be able to calculate derivatives using implicit differentiation.
- Know that the chain rule is the basis for implicit differentiation. [EK 2.1C5]
- Know that the derivative rules we have learned tell us how to do EXPLICIT differentiation. We often use IMPLICIT differentiation in situations where explicit differentiation is difficult or impossible. We do not find an explicit formula for the derivative function, but we do find a way to evaluate derivative values at specific points.
We often use IMPLICIT differentiation in situations where EXPLICIT differentiation (using derivative rules) is difficult or impossible.
|
PRACTICE
TEXTBOOK 3.7
|
|
PRACTICE
KHAN ACADEMY |
SAMPLE CONTENT ASSESSMENT ITEMS
|
| ||||||||||||||||||